
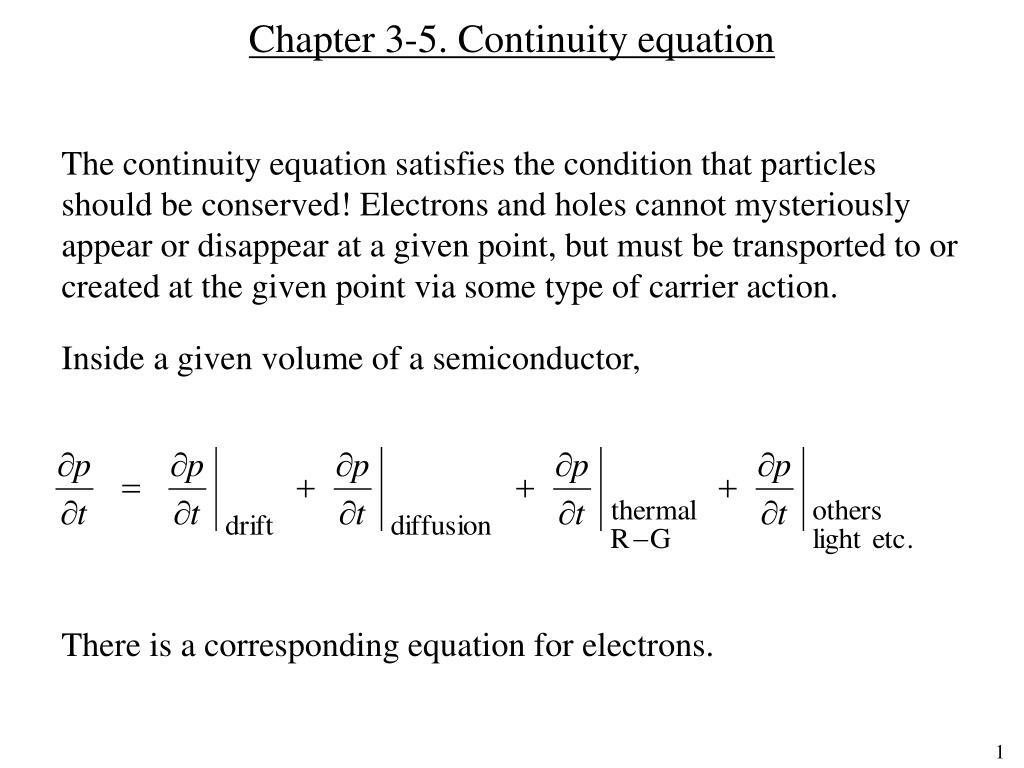
Integrals may be generalized depending on the type of the function as well as the domain over which the integration is performed. In the early 20th century, Henri Lebesgue generalized Riemann's formulation by introducing what is now referred to as the Lebesgue integral it is more robust than Riemann's in the sense that a wider class of functions are Lebesgue-integrable. Bernhard Riemann later gave a rigorous definition of integrals, which is based on a limiting procedure that approximates the area of a curvilinear region by breaking the region into infinitesimally thin vertical slabs. The fundamental theorem of calculus relates definite integrals with differentiation and provides a method to compute the definite integral of a function when its antiderivative is known differentiation and integration are inverse operations.Īlthough methods of calculating areas and volumes dated from ancient Greek mathematics, the principles of integration were formulated independently by Isaac Newton and Gottfried Wilhelm Leibniz in the late 17th century, who thought of the area under a curve as an infinite sum of rectangles of infinitesimal width.

Integrals also refer to the concept of an antiderivative, a function whose derivative is the given function in this case, they are also called indefinite integrals. Conventionally, areas above the horizontal axis of the plane are positive while areas below are negative.
The integrals enumerated here are called definite integrals, which can be interpreted as the signed area of the region in the plane that is bounded by the graph of a given function between two points in the real line. Today integration is used in a wide variety of scientific fields. Integration started as a method to solve problems in mathematics and physics, such as finding the area under a curve, or determining displacement from velocity. Integration, the process of computing an integral, is one of the two fundamental operations of calculus, the other being differentiation. How? Simply click here to return to Your Questions About Limits.In mathematics, an integral is the continuous analog of a sum, which is used to calculate areas, volumes, and their generalizations. Join in and write your own page! It's easy to do. So, applying this with this particular function we have that: This of course is only valid for values "a" in the domain of the function. Why? Because one definition of a continuous function is that:

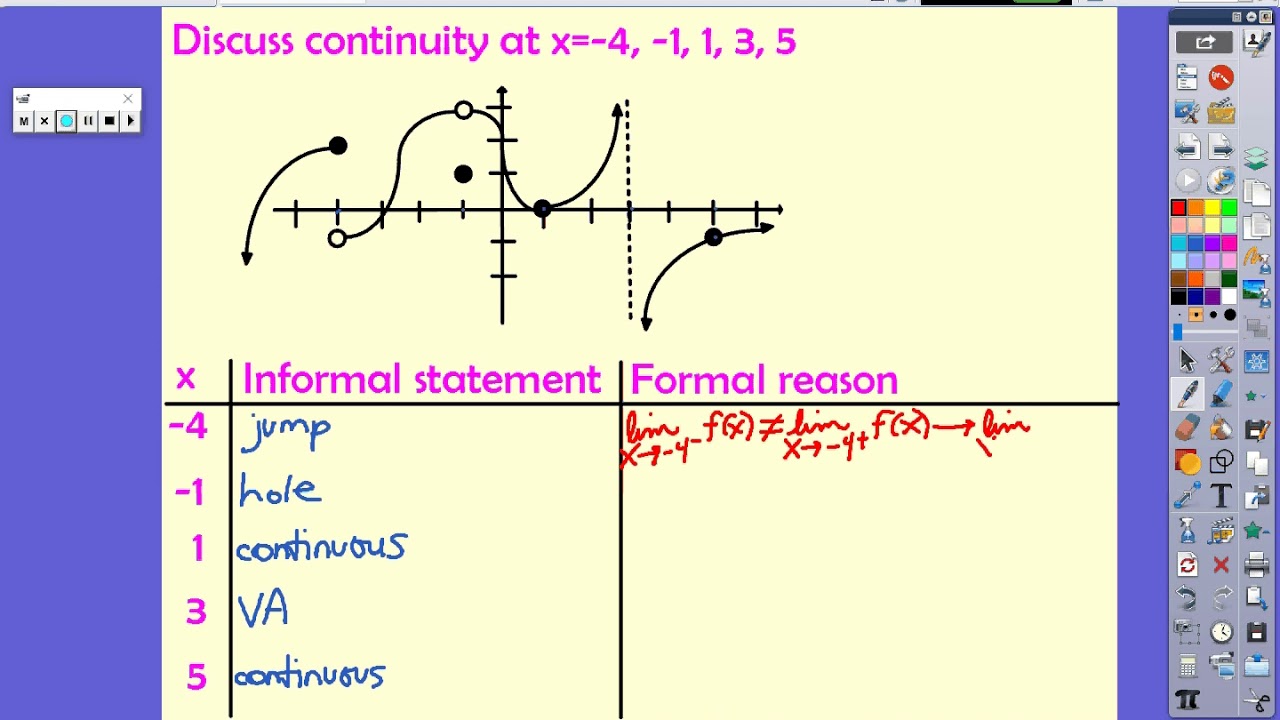
Since these functions are continuous, and this particular function is defined at the point, this value must be the limit. Now, we just need to remember the definition of secant from trigonometry, and the fact that cos(0)=1: In this case, if we evaluate the function at the point we're taking the limit (in this case 0), we get: Usually, the only case where you'll encounter a discontinuous function in these types of problems is when the function is not defined, because the denominator becomes zero somewhere, or we take the square root of a negative number. In this question we have the limit:Īll of these functions are continuous functions. When we're told to solve limits, the first thing we must do is to try to evaluate the function at the point we taking the limit. A Confusing Question to Me: Solving Limits by Continuity


 0 kommentar(er)
0 kommentar(er)
